by Andrew Kliman
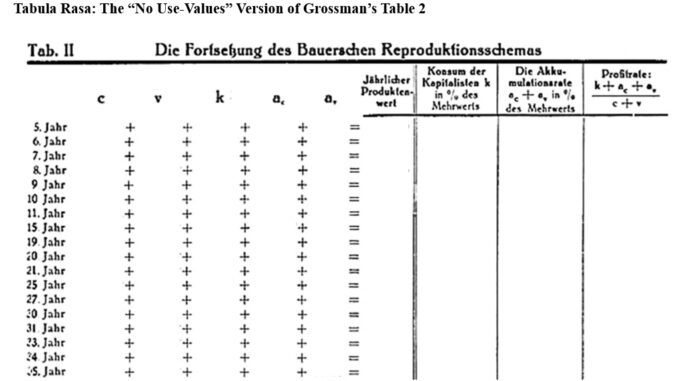
A forthcoming academic paper of mine shows that, contrary to what Henryk Grossman and his followers have consistently claimed, his breakdown tendency and associated phenomena have nothing to do with value or value theory. In his reproduction scheme, the variables have the names of value magnitudes (“surplus-value,” etc.), but this is all value-form, no value substance. The variables’ movements are determined exclusively by movements in physical quantities (of products, means of production, etc.).
A prominent follower of Grossman raised objections (via e-mail) to my demonstrations. He denied that there are any physical (“use-value”) relations implicit in Grossman’s reproduction scheme. He also denied that it is possible to analyze the long-run growth paths of physical variables; because products are continually being discontinued and replaced by other products, physical variables do not have long-run growth paths.
The following article is my reply to these objections. Because the latter part of it uses a lot of mathematical symbols, it is being published as a PDF that you can read in the viewer below or by clicking here.
Editor’s Note, April 13, 2025. The current version of the PDF, uploaded today, corrects and expands on the original version (available upon request). The changes are minor.
Editor’s Note, April 16, 2025. The current version of the PDF, uploaded today, corrects several further errors in notation that were pointed out by Michael in the comments section below.


Hi Andrew,
very interesting food for thought!
But I do have one question.
Shouldn’t equation (7) on page 11
Ct+1 + Vt+1 = ∑ (pjt + Kjt)
be corrected as:
Ct+1 + Vt+1 = ∑ (pjt+1 + Kjt+1)
I.e. the time subscripts t on the right-hand side should be replaced with t+1.
This is because the right and left sides of the equation refer to the same point in time (t+1) and are all “start of the year” variables (as explained in “A. Notation”).
Furthermore, in the second step of equation (9), in the numerator Ct+1 + Vt+1 is effectively replaced by ∑ (pjt+1 + Kjt+1) (and NOT by ∑ (pjt + Kjt)).
This is correct in my opinion, but inconsistent with equation (7) as it currently is.
Thanks so much for your work!
Hi Andrew,
one more thing.
I think in equation (12) the numerator of the sum in the second term must be:
pjt+1 Xjt+1
I.e. the subscript “1” (for kind of product) should be replaced by “j”.
Hi Andrew,
I think I found another minor slip of the pen.
At the bottom of page 19 in text between equation (A4) and (A5) there is a fraction with the denominator ∑ pAt.
But here the price “p” lost its subscript “j”, so the denominator should be ∑ pjAt, i.e. the same as the numerator.
Hi Andrew,
another point:
In whole text A (Physical Quantity of means of production) always appears with a subscript j (for the kind of commodity) and a subscript t or t+1 (for a point in time). And that fine.
But there are – in my opinion – incorrect exceptions:
In the text between equations (A3) and (A4) there is a fraction for ώjt that contains a sum in the denominator ∑ pj At.
A lost here its subscript j.
So, shouldn’t that sum be: ∑ pj Ajt ?
And consequently in the text between (A4) and (A5).
I think it should be:
∑ ώjt = ∑ pj Ajt / ∑ pj Ajt = 1
Then in the formulas before (A6), there is a formula for ώjt.
Here At also “lost” its subscript j.
In (A6) and so on the subscripts are fine afaik.
These subscripts are as annoying as they are necessary.
Hi Michael,
Thanks so much for reading so carefully, and for telling me what needs fixing! I caught the 1st problem (in Eq. (7)) myself, but not the others.
I’ll have a corrected version, fixing the wrong things that you spotted, uploaded soon. As you say, annoying but necessary.
Hi Andrew
I will respond properly when I have the chance. In the mean time you might like to check out this video primer I made on Grossman. Setting the theory aside for a moment, contemporary empirical data is backing Grossman up. Money market funds for example provide a great example of the overaccumulated/surplus capital that proceed a breakdown/recession. As shown around 20-23 mins in the video, they rise steeply before every recession. https://www.youtube.com/watch?v=_VnsnE2dkaU
Best,
Ted
Hi Ted,
In my forthcoming paper (that the article above is about), I address the issue of empirical data:
“Mattick (2018, p. 232, p. 228) construes ‘declining profits, market gluts, and bankruptcies,’ as well as ‘the concentration and centralisation of capital, and the generation of a reserve army of labour’ as evidence that ‘Marx’s [sic] value-theoretic breakdown tendency’ is operative. This is another case in which the evidence disconfirms the existence of Grossman’s breakdown tendency just as much as it confirms it, since it is equally compatible with both Grossman’s and Marx’s theories.
“In addition, the relation of the breakdown theory to most of the phenomena that Mattick mentions is very indirect. Reese’s (2022, chap. 5) discussion of ‘The Final Breakdown’ also construes a slew of very indirectly related phenomena—trends in global debt, declining manufacturing costs, automation, exponential growth of global GDP, etc.—as confirmation of the breakdown theory. It is hard to avoid the conclusion that their interpretations of the evidence are displays of confirmation bias. Would anyone not already committed to Grossman’s breakdown theory interpret the evidence as they do?
“Meaningful empirical tests of the breakdown theory would be ones that test claims and implications of the theory that are unique to it, and that thereby enable us to assess the relative explanatory power of this and alternative theories. And they would test matters much more directly related to the theory, such as the Bauer-Grossman model’s implications that uninvested profit declines in absolute terms and that the ratio of investment to profit and the ratio of consumed constant capital to total value continually rise. I look forward to the results of such tests.”